16. Appendix 1
Calculation in general form of net torque of the device analogous to "Indian wheel"

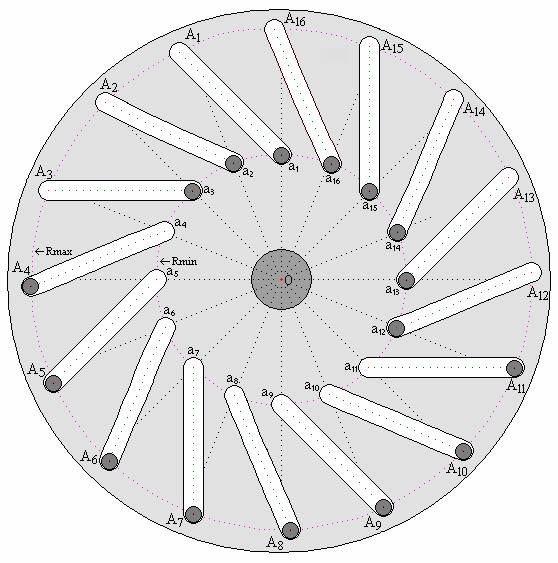
Let us consider again Figure 2, shown in the main text.

Fig. 2

Denotations:

R_min — radius of the circumference, along which are uniformly distributed centers of gravity of movable loads in the state of their minimum distance from the axis of rotation of the disk;

O — center of the disk (the axis of rotation of the disk);

R_max — radius of the circumference, along which are uniformly distributed centers of gravity of the movable loads in the state of their maximum distance from the axis of rotation of the disk (R_max = kR_min, where k – coefficient that indicates the degree of exceeding the length R_max with respect to R_min);

β^°_(Rmin) — angular distance (angle) between the radiuses R_min, outgoing from the center of disk (point “O”) and ingoing in the centers of gravity of two any adjacent loads;

β^°_(Rmax) — angular distance (angle) between the radiuses R_max, outgoing from the center of disk (point “O”) and ingoing in the centers of gravity of two any adjacent loads;

F_g — force of gravity, acting on any movable load;

M_g — torque, generated by a load under the action of only gravitational force;

∑M_g — total torque, produced by all the movable loads under the influence of gravity;

n — total amount of loads.

Initial data for calculation of the device, which corresponds to Figure 2:

1. Total amount of loads — n = 16.
2. The force of gravity acting on any movable load — F_g.
3. The minimum distance of the centers of gravity of loads from the axis of rotation of the disk — R_min.
4. The maximum distance of the centers of gravity of loads from the axis of rotation of the disk — R_max = 2R_min.
5. β^°_(Rmax) = β^°_(Rmin) = 360°/16 = 22.5°.
6. The rotation of the disk counterclockwise accepted as the positive direction of rotation.
7. The angle of incline of the trajectory of the path along which spherical load can slide due to impact of gravity assumed to be 45°. This angle can, for example, be counted (in Fig. 2) as the angle between the axis of the guide tube, starting from the point “a₃” and the ray emanating from the center of the disk (from the point “O”) and passing through the point “a₃”.

We supplement the initial data by introducing Cartesian coordinates, the origin of which is the point “O” (Fig. 2).

Calculation procedure

The torque generated by each load is equal to the product of the gravitational force F_g on the lever arm (d), whose length is determined by the position of the centre gravity of the load relative to the axis of rotation of the disk (point “O” in Fig. 2). The resultant torque is the sum of the torques generated by each load, taking into account their signs. For calculation of the resulting torque (the net torque) is required to calculate the lengths of the lever arms of all loads which are shown in Fig. 2. To do this, first of all, we must calculate the length of the lever arm d, i.e. the coordinate X of the center of gravity of any one load, placed on the circle R_min, and of one - on the circle R_max. The directions of the radii, corresponding to the centres of gravity of two loads, which were originally selected, we take as initial. The calculation of the lengths of the lever arms all other loads we make, taking into account the angles of displacement of the appropriate radiuses (R_min/R_max) relative to the initial directions. The displacement should be done consistently, taking into account that the angles between the directions of any adjacent radiuses are equal to β^° = 22.5°.

Consider the location of the centers of gravity loads shown in Fig. 2. The point a₁ is convenient to take as the starting point for calculation the lengths of the lever arms of torques generated by the loads, whose centers of gravities are from the rotational axis at the distance R_min, and the point A₄ - for the loads at maximum distance from the axis of rotation (at the distance R_max).

The center of gravity of the load a₁ is on the axis Y, passing through the axis of rotation of the disk (through the point “O” ), that corresponds equality to zero for the length of the lever arm (d_a1) of this load (d_a1 = R_min·cos90° = 0).

Calculating the length of the lever arm d_À4, i.e. the coordinate X for the center of gravity of the load A₄, performed in the following order:

1. The coordinate Y is calculated for the point a₃.
   From Fig. 2 it follows that the radius R_min, coming from the axis of rotation of the disk (point “O”) to the point a₃, has been rotated about the axis Y by an angle equal to 2·β^°_(Rmin) = 2·22.5° = + 45°. This enables us to calculate the coordinate Y of the point a₃ in the general form:

   Y_(a3) = R_min·sin45° = 0.701R_min.

2. The coordinate Y is calculated for the point A₃.
   As mentioned above, the radius R_min, coming from the axis of rotation of the disk (point “O”) to the point a₃, is rotated about the axis Y by the angle equal to + 45°. The angle of incline of the trajectory of the path along which spherical load can slide due to gravity assumed to be +45°. Hence, the line a₃ — A₃ is parallel to the axis X. Therefore, the coordinate Y of the point A₃ is equal to the coordinate Y of the point a₃, i.e.

   Y_(A3) = 0.701R_min.

3. The coordinate X is calculated for the point A₃.
   The point A₃ is located on the circumference, the radius of which is R_max. The equation of the circumference in Cartesian coordinates, whose center coincides with the origin of coordinates is: x² + y² = R². By solving this equation for the coordinate X_(A3), we find:

   X_(A3) = √( R²_max − Y²_(A3)) = √(4R²_min − 0.707²R²_min) = R_min√(4 − 0.5) = 1.871R_min.

4. Calculating of the angle between direction of the radius R_max (corresponding to the point A₃) and direction of the coordinate X_(A3):

   ∠φ°_(A3) = arcos(X_(A3)/R_max>) = arcos(1.871R_min/R_max ) = arccos(1.871/2) = 20.69°.

5. Calculating of the angle between direction of the radius R_max (corresponding to the point A₄) and direction of the coordinate X_(A4):

   ∠φ°_(A4) = ∠β°_(Rmax) − ∠φ°_(A3) = 22.5° − 20.69° = 1.81°.

6. The length of the lever arm d_À4, i.e. coordinate X for the center of gravity of the load A₄, is calculated as cathetus of a right triangle with the known length of the hypotenuse R_max = 2R_min and value of adjacent corner ∠φ° _(A4) = 1.81°:

   d_À4 = 2R_min· cos1.81° = + 1.999R_min.

Results of the calculation

The calculation of the net torque produced of all 16 loads was made for the six fixed states of rotation of the disk. The initial state corresponds to Fig. 2. The remaining five states correspond to the results of a consistent rotation of the disk counterclockwise at angles that are listed below:

• β^°_(Rmin)×1/8 = 22.5° ×1/8 = 2.8125°;
• β^°_(Rmin)×1/4 = 22.5° ×1/4 = 5.625°;
• β^°_(Rmin)×1/2 = 22.5°×1/2 = 11.25°;
• β^°_(Rmin)×3/4 = 22.5°×3/4 = 16.875°;
• β^°_(Rmin)×7/8 = 22.5°×7/8 = 19.6875°.

The results of the calculation of the torques provided by each from the 16 loads at these turnings of the disk, and the net torques, corresponding to these states, are shown in Tables 1 and 2. In Table 3 is summarized the results of calculations to assess the behavior of the net torque in the process of turning the disk in the range 0° – 22.5°. Because of the fact that the inclined paths for displacement of the loads are distributed equally and symmetrically about the axis of rotation of disk, the behaviour of the net torque should be repeated in each sector of the turning disk on angle 22.5°.

Findings

The results of calculations show the following.

The rotation of the disk is possible only when another force acts on it. We call it the "external" force (external with respect to gravity). After the cessation of such exposure the disk must to stop. And it should stop in position corresponding to the turning, regarding the state shown in Fig. 2, by the angle β^°_(Rmin)×1/2 = 22.5°×1/2 = 11.25°. And this is entirely consistent with the feature which determined by potential nature of the gravitational field, which consists in the fact that work on the movement of any physical object in a closed path is zero. In the system, corresponding to Fig. 2, the trajectory of the center of gravity of each load - closed. This device, like any other, like it, after the cessation of the external force action, inevitably comes to a state of stable equilibrium^[32]. At this, in the device according to Fig. 2, there are 16 states in which it is possible to ensure of stable equilibrium.

Such a state can be compared with the state of the ball what is shown in the Figure 15.

Fig. 15

The results of calculation in a general form of the net torque allows us to select the magnitude and direction of the optimal annex of external force action to ensure the long lasting rotation of the disk with partial use of the kinetic energy of the gravitational field.

The Figures 2 and 3 of the main text clearly show and the results of calculation (that are listed in Tables 1-3) numerically confirm in the following:

1. The loads, marked in Fig. 2 by the symbols: A₄ – a₁₃, A₅ – a₁₄, A₆ – a₁₅ and A₇ – a₁₆, constitute couples of forces with lever arms of unequal length relative to the axis of disk (point “O” in Fig. 2). They create the total torque of disk directed counter-clockwise.
2. The load in position a₁ does not create a torque drive, since its center of gravity lies on the vertical line passing through the center of the disk “O”.
3. Torques generated by a pair of loads in position a₂ and a₁₆ are mutually cancel each other out, as these loads are located on opposite sides and equidistant from the vertical line perpendicular to the axis of rotation of the disk and passing through the center of the disk axis “O”. The same thing happens with the torques which caused by the loads in position a₃ and a₁₅.
4. Loads A₈, A₉, A₁₀, A₁₁, whose centers of gravity are at the greatest possible distance (R_max) from the axis of rotation of the disk, and the load a₁₂, the center of gravity of which is removed from axis of rotation of the disk at the minimum possible distance (R_min), create negative (clockwise) torque drive that completely compensates the total positive rotatory torque (directed counter-clockwise), created by the loads mentioned in paragraph 1.

*

* * *

It is easy to see, that if by using an external force will be possible to counteract the gravitational impact on the loads, moving in the sector A₈ – O – A₁₁ (Fig. 2), the effect of gravity on this section of the trajectory movement of loads will be reduced to zero. Equilibrium in such a state of the system will be broken. And during the time of action of this external force the resultant rotating effort will be determined by the total torque created by the loads specified in paragraph 1. The disk will be able rotate. At the same time a prerequisite for ensuring the possibility of rotation is that the magnitude of the resulting torque must ensure overcoming the total resistance to the rotation produced by useful load on the motor shaft, friction, heating, and other possible impacts impeding to rotation.

*

* * *

Of course, the bulky calculation, resulted above, does not can add anything new to the fundamental concept of the physics - to the Law of Conservation of Energy, as well as to the feature of a potential character of gravity field of the Earth, concerning the equality to zero of the work of the force acting on a body, moving along the closed trajectory. However, this calculation allows understand, where the most appropriate to apply external force effort, in order to provide in such a system the rotation with partial use of the kinetic energy of the gravitational field of the Earth. The results of calculation "in general form" also allow to obtain numerical estimates of the contribution of each movable load in creating of a common torque by substituting into received symbolic expressions the real numeric values of weight of the movable loads and the values of the corresponding lever arms of rotation.

Successful utilizations of the kinetic energy of the Earth's gravity jointly with the influence of other forces of nature are used by people for a long time. Suffice it to recall, that hydroelectric power plants work by using the kinetic energy of water falling from great heights. But the rise of the water to great heights occurs due to the influence of other forces of nature. Or skier, sliding down to his great satisfaction from the mountains due to the influence of gravity, is forced to use the energy of his muscular effort to rise on mountain.

This page was last modified on 18 September 2014